The efficient solution of the Helmholtz equation
Jok Tang
Site of the project:
Shell
Hoekstede Building
Visseringlaan 26
2280AB Rijswijk
The Netherlands
start of the project: November 2003
In February 2004 the
Interim
Thesis has been appeared.
The Master project has been finished in August 2004
( Masters Thesis).
For working address etc. we refer to our
alumnipage.
Summary of the master project:
The efficient solution of the Helmholtz equation on very large grids
(1000 x 1000 x 1000) is very important for Shell and a challenge for numerical
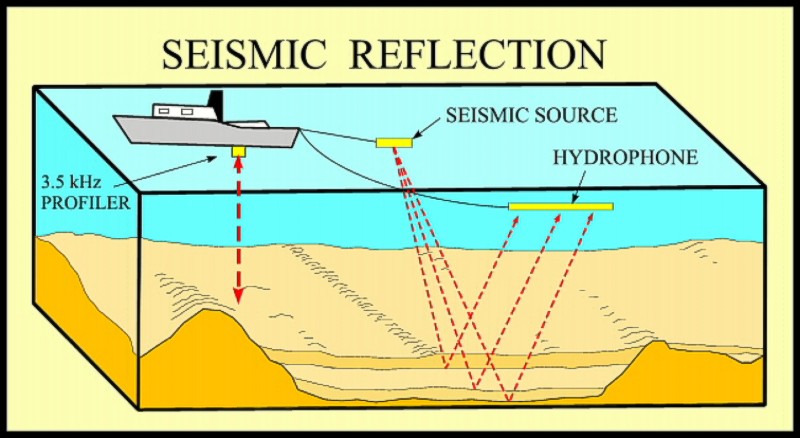
linear algebra research. The Helmholtz equation is used for seismic
investigations of the earth's crust. The results can be used to determine the
position of various layers. Thereafter, possible locations of oil or gas
reservoirs can be predicted.
At this moment a number of solvers are known:
- ILU preconditioners
- multigrid preconditioners
- shifted Laplace preconditioners
- separation of variable preconditioners
- Gander and Nataf preconditioners
Some of these preconditioners work for certain applications, but fail for other
ones (some of which are interesting for Shell). During the master thesis work
literature about these preconditioners should be studied. A comparison of these
methods for realistic problems is important. Investigation of the properties of
the preconditioners can lead to an understanding of their success or failure.
Probably a combination of different preconditioners can lead to a robust and
efficient solver.
To be more specific the following topics can be studied:
- a comparison of method 3 and 4 for realistic 3D problems
- investigate/compare the eigenvalues of the preconditioned matrices for method
3
and 4. For method 3 some idea's are available. Try to prove these idea's
mathematically or by numerical experiment.
- It appears that method 3 has a good behavior for the large eigenvalue
components, but a bad behavior for the small eigenvalue components. If one
of the other methods had a good behavior for the small eigenvalue components
a combination can be very usefull.
- investigation of continuation. Possibilities: increase k or decrease stepsize
or decrease artificial damping etc.

Contact information:
Kees
Vuik

Back to the
home page
or the
Master students page of Kees Vuik


![]()
![]()