An unstructured staggered scheme with quadrilaterals for
the Navier-Stokes equations
( figuren)
Berry van der Veer
Plaats van afstuderen:
TU Delft
start van afstuderen: december 1998
In april 1999 is de
scriptie verschenen.
De afstudeeropdracht is december 1999 afgerond met het
schrijven van het
afstudeerverslag.
Korte omschrijving van de afstudeeropdracht:
Problems in fluid dynamics are often characterized by the Navier-Stokes
equations, which express the conservation of mass, momentum and energy.
In the first part of the report these equations are explained.
In
physics
such equations are usually made dimensionless.
This is done by using a Mach-formulation
for compressible fluids and this method is not suitable
for incompressible fluids.
In reality it is hardly possible to solve the
non-linear Navier-Stokes equations analytically, therefore they will be
treated
numerically. In order to do this we need a discretization.
In the second part of the report the dimensionless equations are
discretized
by the finite volume method. We are making use of a staggered placement
of the
variables on an unstructured grid with quadrilaterals. Finally the
implementation has to be made. This has not been done yet, so in the
concluding
part the steps that need to be done in order to make an implementation
are briefly discussed.
Some figures used in the derivation of the method.
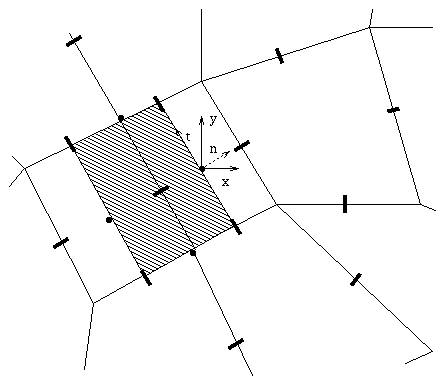
The Cartesian components of the velocity and normal components at the
edges
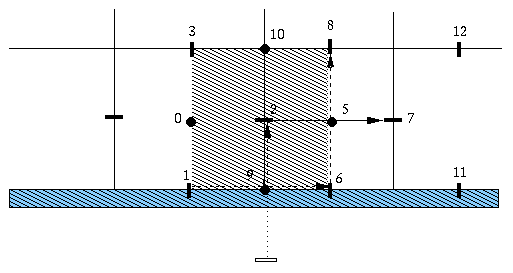
Paths on the edge
Back to
home page
or
Afstudeer pagina
of Kees Vuik


